How to Cite | Publication History | PlumX Article Matrix
A. S. Ahmed1,*, M. E. Abdelwanis1, E. M. Attalla2, Y. Saddeek3 and E. Shaaban3
1Department of radiotherapy and nuclear medicine south Egypt cancer institute Assiut university Assiut Egypt. 2Department of radiotherapy national institute of cancer Cairo university Cairo Egypt 3Department of physics Alazhar university Assiut branch Assiut Egypt *Corresponding other E. mail: ah_farag@hotmail.com
DOI : http://dx.doi.org/10.13005/bbra/2025
ABSTRACT: To compare dosimetric calculation using Clarkson, Convolution, Superposition and Fast Superposition algorithms in Three-Dimensional Conformal Radiotherapy (3D-CRT), and to study the suitability of algorithms with respect to brain tumors and technique. Fifteen brain tumor cases for treatment plans were created using 6 MV Photon beam quality using the CMS XiO (Computerized Medical System, St. Louis, MO) treatment planning system. Patients divided into two sections according to volume of target more or less than 150cc represented by PTV. Six different points were selected to measure the dose. Statistical analysis used: Mean Dose, and Mean Relative Difference have been used Maximum percentage of variation recorded between algorithms was 43.7%, recorded with a surface dose point in case of PTV less than150 cc. The fast superposition algorithm showed excellent results in cases of PTV less than 150ccby considering the mean relative differences with a prescribed dose with four algorithms and minimum relative difference with a prescribed dose in different PTV and treatment techniques. The superposition algorithm showed better results in all techniques. The Clarkson algorithm showed monotonically variation in the dose calculation points and histogram parameters. The other three algorithms are approximately similar in results. Present study has explained the dosimetric results of different algorithms.
KEYWORDS: Algorithm; brain tumors; homogeneity index; treatment planning system
Download this article as:| Copy the following to cite this article: A. S. Ahmed S, Abdelwanis M. E, Attalla E. M, Y. Saddeek Y, Shaaban E. Evaluation of the algorithm’s accuracy in the computation of the dose distribution in the Brain Tumors. Biosci Biotech Res Asia 2016;13(1) |
| Copy the following to cite this URL: A. S. Ahmed S, Abdelwanis M. E, Attalla E. M, Y. Saddeek Y, Shaaban E. Evaluation of the algorithm’s accuracy in the computation of the dose distribution in the Brain Tumors. Biosci Biotech Res Asia 2016;13(1). Available from: https://www.biotech-asia.org/?p=7694 |
Introduction
The functionality and quality of any treatment planning system (TPS) are dependent on the type of algorithms used in the different steps of the planning process. An algorithm is defined as a sequence of instructions that operates on a set of input data, transforming that information into a set of output results that are of interest to the user. Many algorithms are used in the treatment planning process. The most well-known algorithm is the dose calculation algorithm that generates the dose at any point within the patient while taking into account the patient and beam (or source) characteristics[1]. It is of paramount importance for the modern conformal radiotherapy technique to have accuracy in dose calculations in almost all relevant clinical situations. One of these situations is the treatment of lung tumors where irradiation has to be planned under challenging conditions for dose calculation[2]. The accuracy of patient dose predictions has continuously improved by moving from the simple scatter inhomogeneity corrections over pencil beam algorithms to point kernel-based Convolution/Superposition methods [3]. In the present study Clarkson, convolution, superposition, and fastsuperposition algorithms were applied for all plans. The purpose of the present study was to compare the results from four different algorithms, for six different points, representing varied position and heterogeneity conditions and using 3D-CRT“Three-Dimensional Conformal Radiotherapy (3D-CRT)“ Technique. This allowed us to know the suitability of an algorithm for the respective diagnostic and treatment technique. Conformity Index, Homogeneity Index, Mean Dose, and Mean Relative Difference have been used to evaluate the external beam plans. Furthermore, Dose Volume Histograms (DVH) for different structures were obtained to quantify the dose to the other OARs.
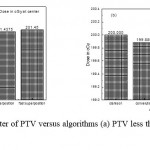 |
Figure 1: Dose in cGy at center of PTV versus algorithms (a) PTV less than 150cc and (b) PTV mor than 150cc. |
Materials and Methods
Patients and techniques
Fifteen Cancer patients with the diagnosis of brain tumors were selected for this study. Doses of 200 cGy/Fraction, were prescribed to the planning target volume of the brain. Planning Target Volume (PTV) was getting drawn by using the 5mm isotropic extension of the Clinical Target Volume (CTV), which in turn was gained from the macroscopic Gross Tumor Volume (GTV). Eight of selected patients have a PTV less than 150 cc, while seven patients have a PTV more than 150 cc. The PTV was made to eliminate other OARs by 5mm.[4] Treatment planning designs for the target and OARs were created using 6MV, 15 MV and mixed 6and15 MV Photon beam quality using Clarkson, convolution, superposition, and fast superposition algorithms. For each patient, six points are selected to compare dose calculation for different algorithms, which used include isocenter of PTV, 3cm from isocenter, 0.5cm under skin, low and high density, and point under the block. Commercially available CMS XiO (Computerized Medical Systems, USA) Planning system was used for planning purposes.
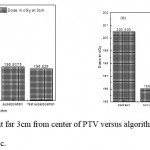 |
Figure 2: Dose in cGy at point far 3cm from center of PTV versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
Dose Calculation Algorithms
Four calculation algorithms used to calculate the radiation dose for all plans which were formed through this study. The XiO’s fast-Fourier transform (FFT) convolution algorithm, and the superposition (Wiesmeyer and Miften) [5]. Algorithms are comparable that, they both calculate the dose by considering the total energy released in the patient with Monte Carlo-generated energy deposition kernels, computed by Mackie et al. [6]. The kernel is the dose matrix created per unit TERMA at the collaborating sites. Total energy released per unit mass (TERMA) produce from the mass attenuation coefficient and the essential energy flounce [7]. The selection of dose calculation algorithms is a serious respect when using “high-ended” planning procedures and comparing one method with another [8–10].
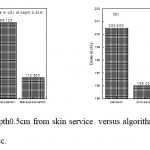 |
Figure 3: Dose in cGy at depth0.5cm from skin service versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
Clarkson Sector Integration Algorithm
One of the most fundamental modified algorithm uses patient data, treatment machine data, and setup information to simulate dose distributions inside the patient. The patient data contain relative electron density information which characterizes a sector of the patient. These have been earlier created by assignment density values in areas incorporated by marking contours or by granting the CT to comparative electron density conversion of patient image data. For a 3-D plan, only transverse patient data are used in the dose computation; Data of The treatment machine required for the Clarkson algorithm must include a set on the diagonal off-center ratios (OCDs), a band of tissue maximum ratios (TXRs) which comes from TAR or modified TPR values, information about the various beam modifiers and different machines (energy) specific constants. If the beam has a multileaf collimator or customized port; the transmissions and outlines of the blocked areas need to be implanted The algorithm takes into account initial dose correction for inhomogeneity in the patient and the transmission by the wedge and bolus, compensators and blocks. Furthermore, the Clarkson algorithm does not take into account scatter modifications due to differences in field intensity (wedges), patient density, or surface curvature. It contracts into account scatter modifications due to field shape. The Clarkson scatter calculation does not accurately the model dose for forked structures because it does not consider the scatter reduction of the air space between the separated contours. Each ray is calculated individually and then the beams are summed together. The full dose of all beams represent the dose that received by the patient [1].
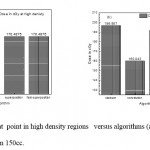 |
Figure 4: Dose in cGy at point in high density regions versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
Convolution Algorithm
The energy deposited kernels of Mackie et al [5]. Must be interpolated from spherical to Cartesian coordinates on a common grid with the TERMA to complete the FFT convolution. Sampling and interpolation of kernels from spherical to Cartesian coordinates are complicated by steep kernel gradients. Adaptive quadrature techniques certify that the correct energy in and near the interaction point is characterized in the Cartesian coordinates.
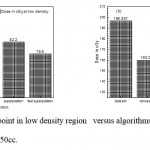 |
Figure 5: Dose in cGy at point in low density region versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
Evaluation of calculation outcomes specifies that incorrect doses are found if the effect of spread from the neighbors is omitted over a large enough area. It is essential that data of the patient be denoted over a 3D volume since the scatter considered as a point is based on the 3D volume of the scattering intermediate. The essential volume over which scatter of kernel contributions must be necessitated and the maximum volume used in XiO planning system is close to 30cm in the forward direction, 5cm in the backward direction, and replicate the field size dimension laterally (basically the contributions from all interaction points must be collected). Sharpe and Battista [11] have reported the same ranges, as well as Mackie et al. [12,13] have described the same obligatory lateral range.
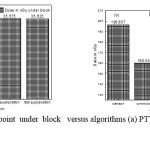 |
Figure 6: Dose in cGy at point under block versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
As well as dose contributions above such a large area needs a significant time for computation. This computation time can be reduced by accomplishment separate calculations; one with the main core for which the computation is done at a high resolution, but over a small region, the other with a scatter kernel where a computation is achieved at a lower resolution but over a large area, as suggested by Mackie et al. [5, 8]. This method is possible for the primary kernels have particularly large gradients close to the point of interaction, but they make no contribution outside a few centimeters from the interaction point, whereas, the scatter kernels have smaller gradients but contribute the dose over a much larger range. The XiO planning system completes a separate high and low resolution FFT calculated for the primary and scatter kernels, saving a time of about 65% over accomplishment a single calculation at high resolution.
 |
Figure 7: Maximum dose in cGy calculated from histogram versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
Superposition Algorithm
The XiO superposition dose accumulated method is a modification of the “collapsed cone” dose calculation process [9]. As with FFT Convolution, all calculations of superposition are done with coordinates of the beam. The dose in the beam coordinates is interpolated to the user quantified calculation volume. It is conceivable for superposition algorithms to directly imitate the kernel calculation method; that is, to compute deposited energy by dispersion the energy released (TERMA) at the collaboration points to points in the interest volume, according to the distribution obscure by the kernel. This method is recognized as the “interaction point of view”. Dissimilar to the FFT convolution algorithms, the superposition algorithm energy deposition kernels can be adjusted to account for fluctuations in electron density. The density scaling method, based on O’Connor’s theorem [14] is applied to distort the kernels by finding the average density along the straight-line path between the interaction site and dose deposition site [15]. A good approximation for scattered photons is Density scaling, because the photons move in straight lines and the mass attenuation coefficient scales linearly with the material density (assuming that the atomic number remains unchanged).
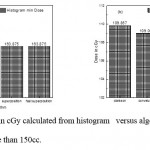 |
Figure 8: Minimum dose in cGy calculated from histogram versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
Fast Superposition Algorithm
Spherical kernels, or “dose spread arrays”, are cylindrically symmetric and well-defined in terms of rays drawn along zenith and azimuth angles. The spherical kernel computation has been enlarged with the ability to combine (select and sum) adjacent zenith rays in the center. Therefore, it is possible to determine the quantity and direction of zenith rays for the function of adjusting speed/accuracy.
Tradeoffs: The less the rays, the quicker and less accurate the calculation, The more the rays, the denser and more accurate the calculation. Although the azimuth angles must be evenly spaced, Control of both the number and direction of zenith rays and azimuth rays is possible,. The fast mode provides a fast superposition dose calculation with a speedup factor of 2.5cm at the monetary value of a small loss in accuracy, compared to the “standard” superposition calculation.
 |
Figure 9: Mean dose in cGy calculated from histogram versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
Dose Reporting and Evaluation
All patients plan calculated to receive 200 cGy through two or more treatment field. Weight values ranging from 1 to 100. The weight applied to decrease or increase the dose over the whole volume of the structure. For each patient, dose volume histograms (DVHs) were produced utilizing the CMS XiO planning system for 3DCRT plan. Single dose-volume point were also registered. Minemum dose, maximum dose, and mean dose were estimated for the PTV and OARs. And Uniformity Index Conformity Index were calculated for PTV in all instances. Relative dose volume differences (percentage) between the outcomes of the different dose calculation algorithms were computed. Maximum percent variation between algorithms were recorded for PTV, for all cases. All the sets of treatment plans were assessed utilizing a lot of evaluation parameters, which complied with the evaluation criteria recommended by the international committee on radiation units and measurements (ICRU) Report 62 [15,16]. The evaluation parameters included the homogeneity index (HI) and the conformity index (CI). The CI was defined as the quotient of the treated volume and the intensity of the PTV [16]. The conventionally used homogeneity index (H-index) is defined as the ratio of the maximum dose in the PTV to the prescribed dose with a value closer to 1 indicating better homogeneity [17]. The H-index commonly varies from 1 to 1.5 in the practical patient treatment plans. The index’s simplicity has led to its being extensively used for quantifying dose homogeneity in tumor volumes. For the evaluation of doses to the OARs, the mean dose was used.
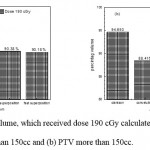 |
Figure 10: Percentage of volume, which received dose 190 cGy calculated from histogram versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
Results and Discussion
As shown in Fig (1.a) we detect insignificant variation in the dosmetric study of the central point of PTV less than 150cc between four algorithms were used. The maximum variation was 0.025%, this ratio increases to 0.06% in cases of target volume more than 150cc see Fig (1.b). Fig (2.a) explain a small variation in dose at a point far 3cm from central point that have a percentage 1.56 of the cases less than 150cc PTV. This variation percentage increase to 2.24 in the cases of PTV more than 150cc, as shown in Fig (2.b). A large change in the doses which calculated at the point have 0.5 cm depth of skin service with PTV less than 150cc cases, where the mean percentage up to 43.7. At the same point, a significant change was detected in the cases of PTV, which more than 150cc with a dose variation percentage 3.5, see Fig. 3.a And 3.b. A significant variations found with inhomogeneity regions represented by points in high intensity (bone) low intensity (air) where the percentage was 5.2 and 3.53, respectively, in the case of PTV less than 150cc. On the other hand, the case of PTV more than 150cc these percentage increase to 18.3 and 5.03 for points in high and low intercity regions see Fig. (4.a), (4.b), (5.a), (5.b). At a point in protected area, we found a large percentage of variation about 12.12, we note in all cases the main variation with doses calculated by Clarkson algorithm and the other three algorithms were relatively close in the case of PTV less than150cc. Same manner were appeared in the case of PTV more than 150cc, but the percentage of variation decrease to 9.7 see figures (6.a, 6.b). Figures (7, 8a) show the maximum and minimum dose of PTV less than 150cc which obtained from histogram calculated by each algorithm, the percentage of variation in maximum dose going to 1.56, this percentage became 4.25 with minimum dose. On the other hand, in case of PTV more than 150cc we found the percentage of variation in maximum dose about 4.14 see Fig.7b, and insignificant in minimum dose which recorded as 0.42% see Fig. 8.b. A small percentage of variation in mean dose which obtained from histogram for each algorithm were recorded about 1.25 and 1.64 in the case of PTV less and more than 150cc, respectively, see Fig. 9a and 9b. Also histogram gives us a percentage of volume, which received 200cGy, and 190cGy, the difference of these percentages founded as 7.78 and 5.35, respectively. In cases of PTV less than 150cc as shown in Fig. 10.a And 11.a. These differences were increased in case of PTV more than 150cc we found a difference in volume percentage, which received 200cGy, and 190cGy at 17.33 and 6.23.
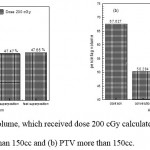 |
Figure 11: Percentage of volume, which received dose 200 cGy calculated from histogram versus algorithms (a) PTV less than 150cc and (b) PTV more than 150cc. |
Conclusion
Dose calculated by four different algorithms understudies gives the same values approximately at center point because no different in field intensity or tissue inhomogeneity at the center. When we are far from the center a considerable percentage of variation appears, as we see in points, which located at three centimeters from the center. This variation was clear with the doses which calculated by Clarkson algorithm due to the Clarkson algorithm does not take into account scatter modifications due to the field intensity (wedges) so this variation increase in the case of PTV more than150cc. At the point of depth of 0.5 cm of the skin surface, large variation appear in dose which calculated with convolution algorithm and superposition algorithm recorded largest value of dose in the case of PTV less than 150cc this Similarity due to both algorithms compute the dose by convolving the total energy released in the patient so they need a large volume of scatter that’s Explains why The variation decrease in the case of PTV more than 150cc.
References
- Kirloskar T. Advantages of multiple algorithms support in treatment planning system for external beam dose calculations. J Cancer Res Ther. 2005;1:12–20.
- Garcia-Vicente F, Minambres A, Jerez I, Modocell I, Perez L, Torres JJ. Experimental validation tests of Fast Fourier Transform convolution and multigrid superposition algorithm for dose calculation in low density media. Radiother Oncol. 2003;67:239–49.
- Vanderstraeten B, Reynaert N, Paelinck L, Madani I, De Wagter C, De Gersem W, et al. Accuracy of patient dose calculation for lung IMRT: A comparison of Monte Carlo,convolution/superposition, and pencil beam computations. Med Phys. 2006;33:31-49.
- De Neve, Wilfried, Yan Wu, and Gary E. “Practical IMRT planning.”Image-guided IMRT. Springer, Berlin Heidelberg, 2006: 47-59.
- Mackie TR, Scrimger JW, Battista JJ. A convolution method of calculating dose for 15 MV X-rays. Med Phys. 1985;12:188–96
- Wiesmeyer MD, Miften MM. A Multigrid approach for accelerating three-dimensional photon dose calculation. Med Phys. 1999;26:11-49.
- Khan FM. The physics of radiation therapy. USA: Lippincot Williams and Wilkins; 2003.
- Beavis AW, Abdel-Hamid A, Upadhyay S. Re-treatment of lung tumor using a simple intensity-modulated radiotherapy approach. Br J Radiol. 2005;78:358-61.
- Miften MM, Beavis AW, Marks LB. The influence of the dose calculation model on Treatment plan evaluation in conformal therapy:A three case study.Med Dosim. 2002;27:51-7.
- Jeraj R, Keall PJ, Siebers JV. The effect of dose calculation accuracy on inverse treatment planning. Phys Med Biol. 2002;47:391–407.
- Sharpe MB, Battista JJ. Dose calculations using convolution and superposition principles: The orientation of dose spread kernels in divergent X-ray beams. Med Phys.1993;20:1685-94
- Mackie TR, El-Kathib E, Battista J, Scrimger JW, Van Dyk J, Cunningham JR. Lung dose corrections for 6-and 15-MV X-rays. Med Phys. 1985;12:327-32.
- Ahnesjö A. Collapsed cone convolution of radiant energy for photon dose calculation in heterogeneous media. Med Phys. 1989;16:577–92.
- Hogstrom KR, Mills MD, Meyer JA, Palta JR, Mellenberg DE, Meoz RT, et al. Dosimetric evaluation of a pencil-beam algorithm for electrons employing a two-dimensional heterogeneity correction. Int J RadiatOncolBiol Phys. 1984;10:561–9.
- Wu VW, Sham JS, Kwong DL. Inverse planning in three-dimensional conformal and intensity- modulated radiotherapy of mid-thoracic esophageal cancer. Br J Radiol 2004;77:568-72.
- Wambersie A, Landberg T, ICRU Report 62: Prescribing Recording and Reporting Photon beam Therapy (Supplement to ICRU Report 50) 1999; Perkins CL, et al. JOP. J Pancreas (Online) 2006;7:372-81.
- Yoon M, Park SY, Shin D, Lee SB, Pyo HR, Kim DY, et al. A new homogeneity index Based on statistical analysis of the dose volume Histogram. J ApplClin Med Phys 2007;8:1-8.

This work is licensed under a Creative Commons Attribution 4.0 International License.





